Small droplet clusters have unusual symmetries absent from colloidal crystals and from large clusters. In our new paper, we discuss the hypothesis that the symmetries of small clusters may be related to an amazing mathematical object, the ADE-classification based on the so-called simply-laced Dynkin diagrams, which are common in several remote areas of mathematics.

This ADE-classification is often illustrated by this graph with p+q+r-2 vertices along the three branches. Only five configurations (p, q, r) are possible, which satisfy the inequality 1/p + 1/q + 1/r > 1, namely, two infinite series (1, 1, n) and (2, 2, n-2) as well as three special cases (2, 3, 3), (2, 3, 4), and (2, 3, 5). These cases correspond to the so-called simply-laced Dynkin diagrams (called in the group theory An, Dn, E6, E7, and E8), which classify various mathematical objects. Thus, if (p, q, r) is understood as the rotational symmetry around a vertex (p), edge (q), and face (r), than E6, E7, and E8 represent the symmetries of platonic polyhedral: tetrahedron (2,3,3), cube/octahedron (2,3,4), and dodecahedron/icosahedron (2,3,5), while An represents a flat n-edged polygon (1,1,n), and Dn represents a two-sided flat polygon with n-2 edges (2,2,n-2).

The ADE-classification by the simply-laced Dynkin diagrams involves two infinite series of objects and three special cases, which quickly saturate. This configuration has been suggested for several dozens of diverse mathematical objects ranging from platonic solids to singularities of algebraic hypersurfaces, to critical points of functions having no moduli, and to sophisticated objects in the string theory. V. I. Arnold called attention to the need to understand deep connections between remote areas of mathematics, where the ADE-classification emerges.

In a sense, the ADE-classification is a shadow in our world of some symmetries in idealized mathematical worlds very far away, such as the 248-dimensional or even 196,883-dimensional spaces.

For our small droplet clusters, we also have two infinite series of symmetric clusters and three short series which saturate quickly. Can it be that we have discovered a new example of the ADE-classified object? Please read about it in our, just accepted, paper.

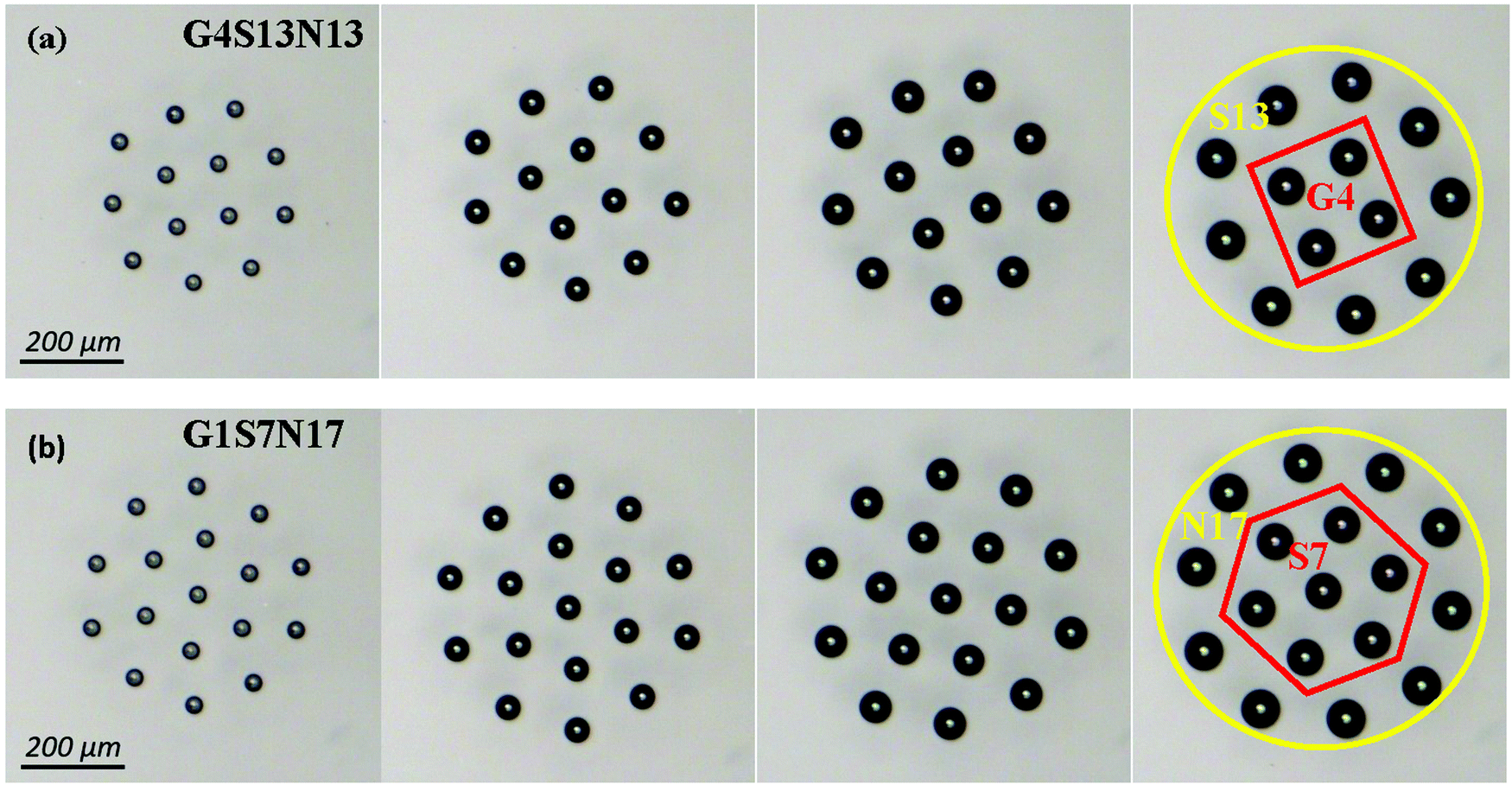
A. Fedorets, E. Bormashenko, L. Dombrovsky, and M. Nosonovsky “Symmetry of small clusters of levitating water droplets” Phys. Chem Chem. Phys. https://doi.org/10.1039/D0CP01804J