| 12/11 |
- Read (or finish reading) Section 6.5 (The Weierstrass Factorization Theorem) for discussion on Thursday. You may read the whole section if you wish, but it will be sufficient to go only as far as Theorem 5.14 (the case where
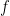 is an entire function). is an entire function).
|
| 12/06 |
- Read Section 6.2 (Spaces of Analytic Functions) and as much as you can of Section 6.5 (The Weierstrass Factorization Theorem) for discussion next week.
|
| 12/04 |
- Finish reading Section 7.1 (The Space of Continuous Functions
 ) for discussion on Thursday ) for discussion on Thursday
- Work on the final exam. You may consult with your classmates as you complete the exam, but only with them. The paper you hand in must ultimately be your own work, and written up by you alone. Due 12/18.
|
| 11/29 |
- Read Section 7.1 (The Space of Continuous Functions
 ) for discussion on Tuesday. This is a long section, so don’t worry if you don’t finish it completely, but go as far as you can in order to save yourself reading time next week. ) for discussion on Tuesday. This is a long section, so don’t worry if you don’t finish it completely, but go as far as you can in order to save yourself reading time next week.
- From Conway, Section 6.4: 2, 3, 7. (Verify that the approach to the first part of Exercise 3 that we took in class was unnecessarily complicated: if we are prepared to consider
 , rather than just , rather than just  , we may as well take , we may as well take  .) .)
- Hand in Section 6.4: 3. Due 12/06.
|
| 11/27 |
- Read Section 6.4 (The Phragmen-Lindelof Theorem) for discussion on Thursday.
- From Conway, Section 6.3: 2, 3, 4, 6.
|
| 11/20 |
- Have a good Thanksgiving!
|
| 11/15 |
- Read Section 6.3 (Convex Functions and Hadamard’s Three Circles Theorem) for discussion on Tuesday.
- From Conway, Section 6.2: 1, 2, 3, 4, 5.
- Hand in Section 6.1: 4; Section 6.2: 3, 4. Due 11/20.
|
| 11/13 |
- Read Sections 6.1 (The Maximum Principle) and 6.2 (Schwarz’s Lemma) for discussion on Thursday.
- From Conway, Section 5.2: 12, 13; Section 5.3: 6, 8; Section 6.1: 3, 4, 6, 7, 8.
|
| 10/30 |
- Read Section 5.3 (The Argument Principle) for discussion in class on Thursday.
- Work on the midterm exam. You may consult with your classmates as you complete the exam, but only with them. The paper you hand in must ultimately be your own work, and written up by you alone. Due 11/08.
|
| 10/25 |
- Read Section 5.2 (Residues) for discussion in class on Tuesday.
- From Conway, Section 5.1: 6, 7, 8, 9, 13.
|
| 10/23 |
- Finish reading Section 5.1 (Classification of Singularities) for discussion in class on Thursday.
- From Conway, Section 5.1: 1(a)(b)(g)(h)(j), 2, 3.
|
| 10/18 |
- Start reading Section 5.1 (Classification of Singularities) for discussion in class next week. (The readings are getting slightly ahead of the class discussion, so don’t feel you need to read all of this section before Tuesday, but my current plan is to try and finish it by next Thursday.) Send me a question, on this reading or anything ealier, by Monday evening. Due 10/22.
- Hand in Section 4.7: 2, 7. Due 10/25.
|
| 10/16 |
- Read Section 4.8 (Goursat’s Theorem) for discussion in class on Thursday.
- Look back over Chapter 4, and see if you have any remaining questions on the material in this chapter. Send me an e-mail with anything you would still like to discuss in class.
- From Conway, Section 4.7: 1 (although Conway did prove this result earlier), 2, 3, 4, 7.
|
| 10/11 |
- Read Section 4.7 (Counting Zeros; the Open Mapping Theorem) for discussion in class next Tuesday. Send me a question on the reading by Monday evening. Due 10/15.
- From Conway, Section 4.5: 1, 3, 4, 8, 10.
- Hand in Section 4.4: 3; Section 4.5: 8. Due 10/18.
|
| 10/04 |
- Read Sections 4.4 (The Index of a Closed Curve) and 4.5 (Cauchy’s Theorem and Integral Formula) for discussion in class next Thursday. Send me a question on the reading by Wednesday evening. Due 10/10.
- From Conway, Section 4.3: 1, 3, 7, 8, 9, 10.
- From Conway, Section 4.4: 1, 2, 3, 4.
- I will not be in class next Tuesday, October 9. Work together during our regular class time to solve Exercises 4.3: 7, 9 and Exercises 4.4: 2, 4.
- Hand in Section 4.3: 9; Section 4.4: 1. Due 10/11.
|
| 10/02 |
- Read Section 4.3 (Zeros of an Analytic Function). You don’t have to send me a question this time, but feel free to do so if there is anything you would particularly like to have us discuss on Thursday.
- From Conway, Section 4.2: 1, 2, 3, 4, 6, 7, 9(a)(c), 13, 14.
|
| 09/27 |
- Read Section 4.2 (Power Series Representation of Analytic Functions) and be awed! (This section tells you just how special analytic functions really are.) Send me a question on the reading by Monday evening. Due 10/01.
- From Conway, Section 4.1: 3, 4, 5, 8, 15, 16, 21, 22, 23.
- Hand in Section 4.1: 12, 22. Due 10/04.
|
| 09/25 |
- Re-read Section 4.1 (Riemann-Stieltjes Integrals). You don’t have to send me a question this time, but feel free to do so if there is anything you would particularly like to have us discuss on Thursday.
- Try to complete the first 3 questions on the “Functions of Bounded Variation” worksheet (on the “Notes” page). These are quite similar to Conway’s Proposition 1.2, and his Exercises 1 and 2.
- From Conway, Section 4.1: 1, 2, 6, 7, 9, 10, 11, 12, 14, 19.
|
| 09/20 |
- Read Section 4.1 (Riemann-Stieltjes Integrals). Send me a question on the reading by Monday evening. Due 09/24.
- From Conway, Section 3.3: 17, 21, 22, 23, 24, 25, 26.
- Hand in Section 3.3: 17, 26. Due 09/27.
|
| 09/18 |
- No new reading for today, but feel free to re-read Section 3.3 in light of today’s discussion and see if any of it is clearer now (or if you have any new questions).
- From Conway, Section 3.3: 1, 4, 6, 8, 9, 14, 15, 20.
|
| 09/13 |
- Read Section 3.3 (Analytic Functions as Mappings. Möbius Transformations). Send me a question on the reading by Monday evening. Due 09/17.
- From Conway, Section 3.2: 1, 2, 3, 6, 7, 10, 14.
- Hand in Section 3.2: 2, 14. Due 09/20.
|
| 09/11 |
- From Conway, Section 2.4: 2, 3, 5.
- From Conway, Section 3.1: 1, 3, 5, 6, 7.
- Read Section 3.2 (Analytic Functions). This is a long section, but you should find much of it to be familiar by now.
|
| 09/06 |
- From Conway, Chapter 1: Section 1.4: 3; Section 1.5: 1; Section 1.6: 1.
- From Conway, Chapter 2: Section 2.2: 3.
- Read Section 3.1 (Power Series), and send me a question by Monday afternoon. Due 09/10.
- (Optional!) If you want to get ahead with the reading over the weekend, feel free to read Section 3.2 (Analytic Functions).
|
| 09/04 |
- Look through Chapters 1 and 2 of the textbook quickly (but concentrating on definitions and on statements of theorems), and make notes of topics and/or material that you would like to review at some point in the semester (Note that we may review material as we need it, not all at once.) Send me your list of topics by noon on Thursday. Due 09/06.
|